Artist’s Statement
I do not recall the exact moment when I decided to become a mathematical artist. It must have been around the summer of 2014, when I purchased a copy of a book titled “ M.C. Escher: the graphic work” [1] while on a vacation in Northern Michigan. This time my interest exceeded the initial fascination about tessellations and optical illusions, which started ten years earlier, and I began to believe that I could produce art of the same genre. Prior to that my artistic production was limited to a small number of pencil sketches and watercolors completed sporadically through the years and mostly during my spare time.
My interest in graphic art started when my father introduced me at an early age to the monumental work “Description de l’Égypte”. I recall spending hours copying the engravings detailing flora, fauna, rocks, archeological sites and modern architectures encountered by the scientific members of Napoleon’s military campaign in Egypt during the late eighteenth century. What fascinated me the most about the engravings was the economic depiction of organic, inorganic, and geometric forms by various degrees of shading and cross hatching. In these illustrations, the connection between art and science was clear although indirect.
I rarely know the subject of my artwork in advance and I find it extremely difficult to plan a theme in a systematic fashion. I begin by exploring the potential of different mathematical ideas and concepts in a playful fashion, without prematurely translating them into an artwork. I also explore different concepts in optical illusions, visual psychology, biology, computer graphics, etc. I trust that some of these concepts will click together every once in a while and in a very organic way. This ‘click’ of ideas and concepts manifests itself in a clear, mental image, which I can use to guide my systematic work of translating ideas first to vector graphics and eventually to paper.
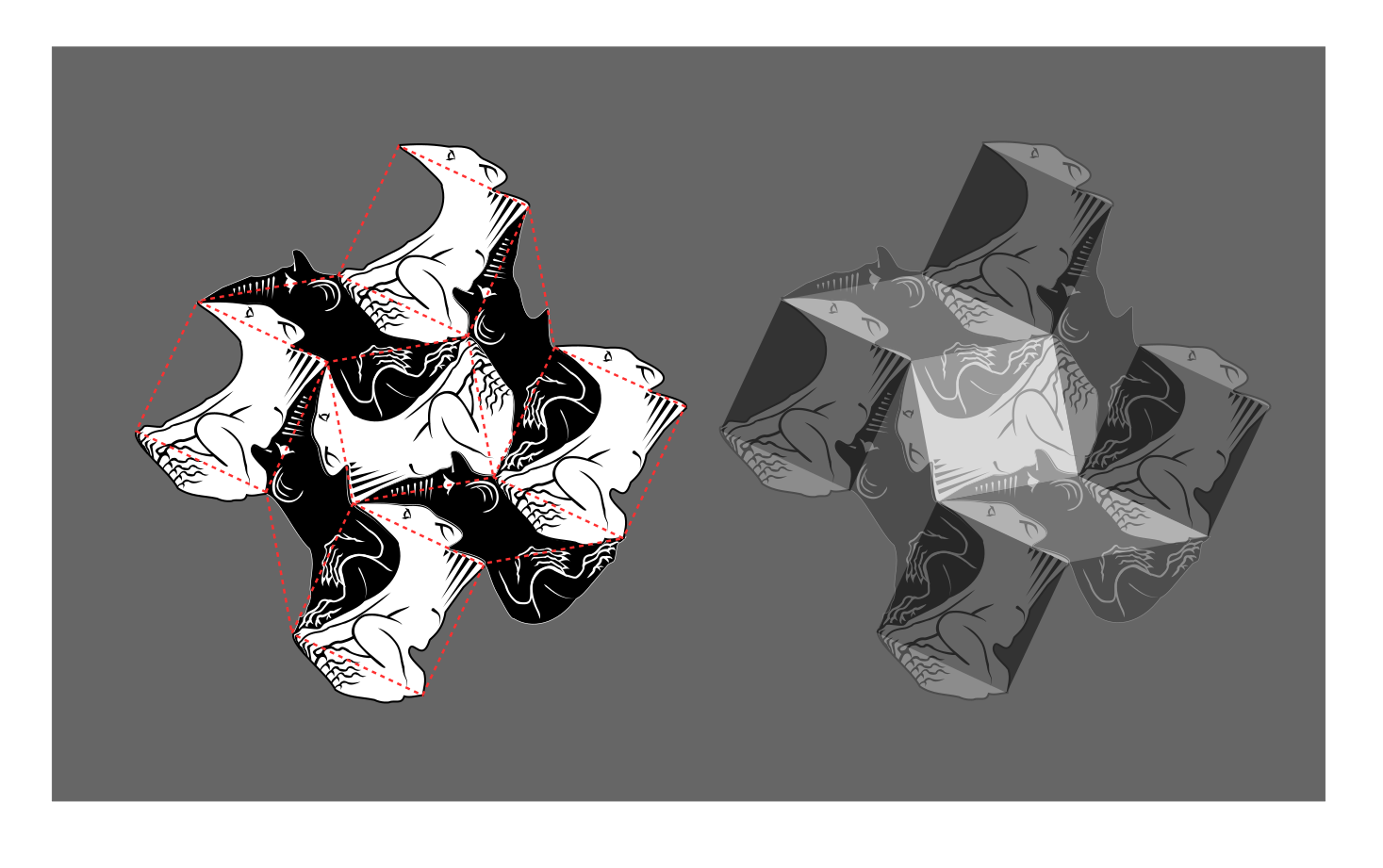
My main artistic strategy depends on the concept of layering. Take for example my artwork titled “Folded Snub Tessellation”. The artwork is based on a modified snub square tiling. This is a classical semi-regular tessellation that has been well-known for more than four hundred years and was described by Johannes Kepler in his book Harmonices Mundi [2]. I used this tessellation as a base for creating two congruent, grotesque motifs, a winged, and horned monster. The two monsters fill the planar space completely without leaving any gaps and require two colors to contrast their shapes.
It also happens that the same semi-regular tile could be interpreted three dimensionally. The rhombic shapes in the tile gives an optical illusion of an isometric projection compared to the surrounding square tiles. I emphasized this perspective by shading the titles to give the illusion of an illumination from a single source of light. The final graphic work that you see is achieved by layering the two-monsters tile over the shaded one. I added an identical copy of the design without shading the tile to demonstrate that the three dimensional effects are an optical illusion.
References
[1] M.C. Escher: the graphic work. Taschen, 2000.
[2] J. Kepler, Harmonices Mundi. Johann Planck, 1619.